Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规初等交换子
,若G存在正规初等交换子 ,则G是不可分辨的.
,则G是不可分辨的.
 ;[数]交换
;[数]交换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规初等交换子
,若G存在正规初等交换子 ,则G是不可分辨的.
,则G是不可分辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系数矩阵和极大项,证明了这类李代数是半单李代数且没有二维交换子代数。
声明:以上例句、词性分类均由
 资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
 换的(阿贝尔是挪威数学家)
换的(阿贝尔是挪威数学家) ;[数]
;[数] 换
换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规
,若G存在正规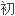
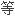
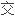 换子
换子 ,则G是
,则G是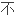

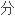 辨的.
辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系数矩阵和极大项,证明了这类李代数是半单李代数且没有二维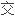 换子代数。
换子代数。
声明:以上例句、词性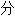 类均由互联网资源自动生成,部
类均由互联网资源自动生成,部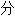 未经过人工审核,其表达内容亦
未经过人工审核,其表达内容亦 代表本软件的观点;若发现问题,欢迎向我们指正。
代表本软件的观点;若发现问题,欢迎向我们指正。
 ;[数]交换
;[数]交换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规初等交换子
,若G存在正规初等交换子 ,则G是不可分辨的.
,则G是不可分辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系数矩阵和极
 ,
, 明了这类李代数是半单李代数且没有二维交换子代数。
明了这类李代数是半单李代数且没有二维交换子代数。
声明:以上
 、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
 换的(阿贝尔是挪威数学家)
换的(阿贝尔是挪威数学家) ;[数]
;[数] 换
换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规
,若G存在正规
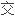 换子
换子 ,则G是
,则G是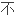

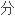 辨的.
辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系数矩阵和极大项,证明了这类李代数是半单李代数且没有二维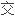 换子代数。
换子代数。
声明:以上例句、词性 类均由互联网资源自动生成,部
类均由互联网资源自动生成,部 未经过人工审核,其表达内容亦
未经过人工审核,其表达内容亦 代表本软件的观点;若发现问题,欢迎向我们指正。
代表本软件的观点;若发现问题,欢迎向我们指正。
 换的(阿贝尔是挪威数学家)
换的(阿贝尔是挪威数学家) ;[数]
;[数]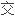 换
换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规
,若G存在正规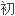
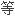
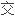 换子
换子 ,则G是
,则G是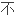

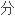 辨的.
辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系数矩阵和极大项,证明了这类李代数是半单李代数且没有二维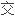 换子代数。
换子代数。
声明:以上例句、词性 类均由互联网资源自动生成,部
类均由互联网资源自动生成,部 未经过人工审核,其表达内容亦
未经过人工审核,其表达内容亦 代表本软件的观点;若发现问题,欢迎向我们指正。
代表本软件的观点;若发现问题,欢迎向我们指正。
 的(阿贝尔是
的(阿贝尔是
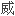
 学家)
学家) ;[
;[ ]
]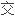
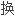

Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规初等
,若G存在正规初等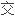
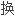
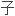
 ,则G是不可分辨的.
,则G是不可分辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系 矩阵和极大项,证明了这类李代
矩阵和极大项,证明了这类李代 是半单李代
是半单李代 且没有二维
且没有二维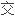
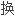
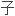 代
代 。
。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。
 学家)
学家) ;[
;[ ]交换
]交换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是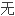

 ,若G存在正规初等交换子
,若G存在正规初等交换子 ,则G是不可分辨的.
,则G是不可分辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系
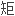
 和极大项,证明了这类李代
和极大项,证明了这类李代 是半单李代
是半单李代 且没有二维交换子代
且没有二维交换子代 。
。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。

 )
) ;[数]交换
;[数]交换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G是无限 ,若G存在正规初等交换子
,若G存在正规初等交换子 ,则G是不可分辨的.
,则G是不可分辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系数矩阵和极大项,证明了这类李代数是半单李代数且没有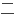
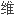 交换子代数。
交换子代数。
声明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不代表本软件的观点;若发现问题,欢迎向我们指正。

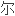 的,交换的(阿
的,交换的(阿
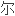
 挪威
挪威 学家)
学家)
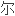
 ;[
;[ ]交换
]交换
Let G be an infinite group. If G has a normal elementary abelian subgroup, then G is irrecognizable.
设G 无限
无限 ,若G存在正规初等交换子
,若G存在正规初等交换子 ,则G
,则G 不可分辨的.
不可分辨的.
Using the notion of coefficient matrix and maximal element.We prove that the Lie algebra is semi-simple and it has no abelian two dimensional subalgebra.
利用系 矩阵和极大项,证明了这类李
矩阵和极大项,证明了这类李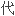

 半单李
半单李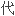
 且没有二维交换子
且没有二维交换子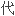
 。
。
 明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不
明:以上例句、词性分类均由互联网资源自动生成,部分未经过人工审核,其表达内容亦不 表本软件的观点;若发现问题,欢迎向我们指正。
表本软件的观点;若发现问题,欢迎向我们指正。